Research interests and presentations
Currently, I am focusing on the structure and the stabilization mechanism of quasicrystalline compounds. I use several different approaches, such as ab initio method, mathematical modeling techniques, etc.
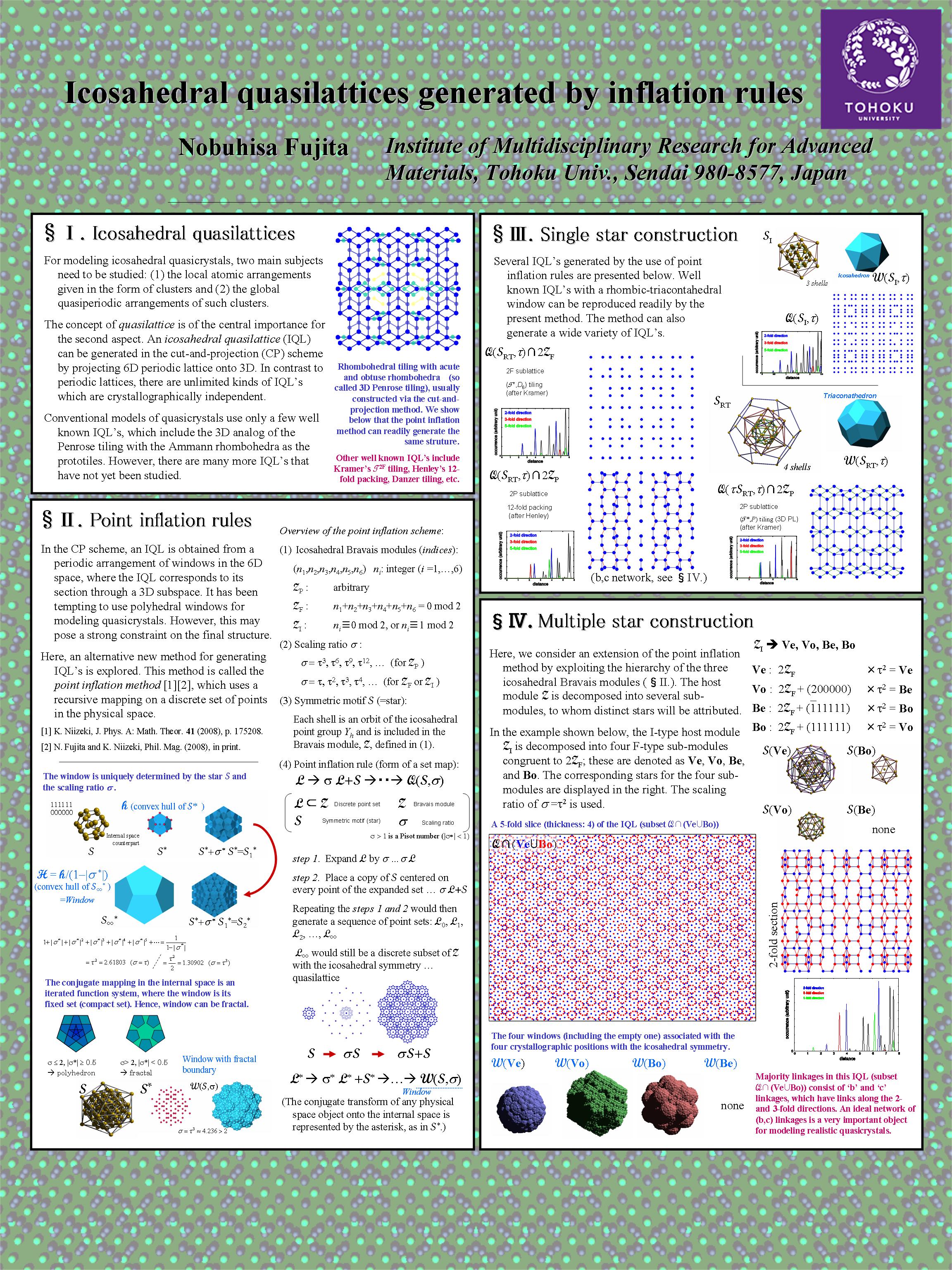
Quasicrystals
A poster that was presented at the anual research meeting of our instituteDecember 12, 2007, Sendai, Japan

A poster that was presented at IUCr 2008 (XXI Congress and GeneraL Assembly of the International Union of Crystallography)
August 23-31, 2008, Osaka, Japan
Two seminars given at the Dept. Phys., Kinki University (in c/o Dr T. Dotera)
January 13-14, 2010, Kinki University, Higashi-Osaka, Japan
Seminar abstracts (PDF) (in Japanese)
Seminar (I) (slides in PDF) : this talk (in Japanese) is about my recent work (Acta Cryst. A 65, 342-351 (2009); Journal of Physics: Conference Series, accepted for publication) on quasiperiodic tilings.
Seminar (II) (slides in PDF) : this talk (in Japanese) is about my previous paper (Phys. Rev. B 72, 085459 (2005)) on band structures of triply periodic minimal surfaces.
JPS 2010 Spring Meeting (The 65th JPS Annual Meeting)
Mar.20th(sat.)-23rd(tue.), 2010, Okayama University (Tsushima Campus)
Invited talk (slides in PDF) (in Japanese)
This talk (in Japanese) is given at Symposium on "What's in between periodicity and non-periodicity: physics of quasiperiodicity" (Mar.20)
11th International Conference on Quasicrystals (ICQ11),
Satellite Meeting on Mathematics of Quasi-Periodic Order
ICQ11: June 13th(sun.)-18th(fri.), 2010, Hokkaido University (Sapporo)
Satellite: June 21st(mon.)-23rd(wed.), 2010, RIMS, Kyoto University
Talk (slides in PDF)(in English)
Computer programs: drawing quasiperiodic tilings in the plane
(1) Program 1: AmmannBeenker2pov.pl
(2) Program 2: Penrose2pov.pl
These programs (written in Perl, approx. 300 lines) generate Ammann-Beenker and rhombic Penrose tilings with the grid method. To run with Windows, you need ActivePerl (ver 5.12) and Povray (v.3.6) installed on your computer. These can be downloaded from
http://www.activestate.com/activeperl/downloads
http://www.povray.org/download
The installation is very simple.
Put each of the .pl files into an empty folder. Double clicking the file to run. Then three output files are generated. (The '.pl' extension should be associated with perl command. But usually it is done automatically when you install ActivePerl.) The output files are (1) the tiling, (2) grid lines (3) mapping of the vertices to the internal space. If you open them in Povray and run, each of these objects can be displayed.
The International School on Aperiodic Crystals. (homepage)
26 September -- 2 October 2010 in Carqueiranne, France.
Lecture on "Aperiodic Tilings" (slides: =1= and =2=)
Slides for all the lectures
さきがけ数学塾「変分法入門」(2011年3月7‐9日,東京)で行った講演
「極小曲面を用いた量子ナノ構造」 (slides;lecture notes)
Personal data from the database of our institute
Last updated on March 11, 2011.
Copyright, 2011 Nobuhisa Fujita, All rights reserved.